Electromagnetic Induction. Maxwells Equations
Problem 3.302
A conducting rod of mass slides without friction over two long conducting rails separated by a distance (Fig. 3.88). At the left end the rails are interconnected by a resistance The system is located in a uniform magnetic field perpendicular to the plane of the loop. At the moment the rod starts moving to the right with an initial velocity Neglecting the resistances of the rails and the rod as well as the self-inductance, find: (a) the distance covered by the rod until it comes to a standstill; (b) the amount of heat generated in the resistance during this process.
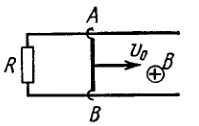
(a) (b)