Problem 3.303

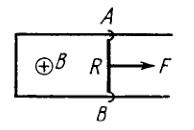
A connector $A B$ can slide without friction along a \PiIshaped conductor located in a horizontal plane (Fig. 3.89). The connector has a length $l,$ mass $m,$ and resistance $R .$ The whole system is located in a uniform magnetic field of induction $B$ directed vertically. At the moment $t=0$ a constant horizontal force $F$ starts acting on the connector shifting it translationwise to the right. Find how the velocity of the connector varies with time $t .$ The inductance of the loop and the resistance of the Tl-shaped conductor are assumed to be negligible.