Electromagnetic Induction. Maxwells Equations
Problem 3.292
A wire loop enclosing a semi-circle of radius \(a\) is located on the boundary of a uniform magnetic field of induction \(B\) (Fig. 3.80). At the moment \(t=0\) the loop is set into rotation with a constant angular acceleration \(\beta\) about an axis \(O\) coinciding with a line of vector \(\mathbf{B}\) on the boundary. Find the emf induced in the loop as a function of time \(t\). Draw the approximate plot of this function. The arrow in the figure shows the emf direction taken to be positive.v
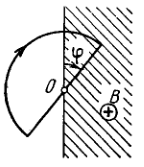
3.292. \(\mathscr{E}_{i}=1 / 2(-1)^{n} \operatorname{Ba} \beta t,\) where \(n=1,2, \ldots\) is the num- ber of the half-revolution that the loop performs at the given moment \(t\). The plot \(\mathscr{E}_{i}(t)\) is shown in Fig. 25 where \(t_{n}=\sqrt{2 \pi n / \beta}\).