All Problems

Electric Current

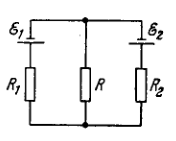
Problem 3.197

In a circuit shown in Fig. 3.54 resistances \(R_{1}\) and \(R_{2}\) are known, as well as emf's \(\mathscr{E}_ {1}\) and \(\mathscr{E}_{2} .\) The internal resistances of the sources are negligible. At what value of the resistance \(R\) will the thermal power generated in it be the highest? What is it equal to?

Reveal Answer
 3.197. R=R1R2/(R1+R2)Qmax⁡=(E1R2+E2R1)2/4R1R2(R1+R2).\begin{array}{c} \text { 3.197. } R=R_{1} R_{2} /\left(R_{1}+R_{2}\right) \\ Q_{\max }=\left(\mathscr{E}_{1} R_{2}+\mathscr{E}_{2} R_{1}\right)^{2} / 4 R_{1} R_{2}\left(R_{1}+R_{2}\right) . \end{array}
Prev
Next