All Problems

The Fundamental Equation of Dynamics

Problem 1.61

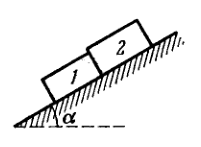
Two touching bars 1 and 2 are placed on an inclined plane forming an angle \(\alpha\) with the horizontal (Fig. 1.10). The masses of the bars are equal to \(m_{1}\) and \(m_{2},\) and the coefficients of friction between the inclined plane and these bars are equal to \(k_{1}\) and \(k_{2}\) respectively, with \(k_{1}>k_{2} .\) Find: (a) the force of interaction of the bars in the process of motion; (b) the minimum value of the angle \(\alpha\) at which the bars start sliding down.

Reveal Answer
 (a) F=(k1−k2)m1m2gcos⁡αm1+m3; (b) tan⁡αmin⁡=k1m1+k2m2m1+m2\text { (a) } F=\frac{\left(k_{1}-k_{2}\right) m_{1} m_{2} g \cos \alpha}{m_{1}+m_{3}}; \text { (b) } \tan \alpha_{\min }=\frac{k_{1} m_{1}+k_{2} m_{2}}{m_{1}+m_{2}}
Prev
Next