Problem 1.257

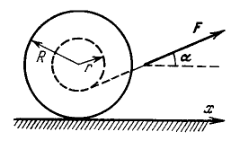
A spool with thread wound on it, of \(\mathrm{mass} m,\) rests on a rough horizontal surface. Its moment of inertia relative to its own axis is equal to \(I=\gamma m R^{2},\) where \(\gamma\) is a numerical factor, and \(R\) is the outside radius of the spool. The radius of the wound thread layer is equal to \(r\). The spool is pulled without sliding by the thread with a constant force \(F\) directed at an angle \(\alpha\) to the horizontal (Fig. 1.63). Find: (a) the projection of the acceleration vector of the spool axis on the \(x\) -axis; (b) the work performed by the force \(\mathbf{F}\) during the first \(t\) seconds after the beginning of motion.