Problem 1.243

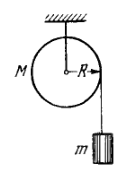
A light thread with a body of mass $m$ tied to its end is wound on a uniform solid cylinder of mass $M$ and radius $R$ (Fig. 1.55). At a moment $t=0$ the system is set in motion. Assuming the friction in the axle of the cylinder to be negligible, find the time dependence of (a) the angular velocity of the cylinder; (b) the kinetic energy of the whole system.